Avogadro's law (sometimes referred to as Avogadro's hypothesis or Avogadro's principle) or Avogadro-Ampère's hypothesis is an experimental gas law relating the volume of a gas to the amount of substance of gas present.^[1] The law is a specific case of the ideal gas law. A modern statement is:

1. Avogadro's Law Is Expressed As
2. Avogadro's Law Chemistry
3. How To Use Avogadro's Number

Avogadro’s law is in evidence whenever you blow up a balloon. The volume of the balloon increases as you add moles of gas to the balloon by blowing it up. If the container holding the gas is rigid rather than flexible, pressure can be substituted for volume in Avogadro’s law. Adding gas to a rigid container makes the pressure increase. Avogadro's law is also known as Avogadro's hypothesis or Avogadro's principle. The law dictates the relationship between the volume of a gas to the number of molecules the gas possesses. This law like Boyle's law, Charles's law, and Gay-Lussac's law is a specific case of the ideal gas law. This law is named after Italian scientist Amedeo Avogadro.

Avogadro's law states that 'equal volumes of all gases, at the same temperature and pressure, have the same number of molecules.'^[1]

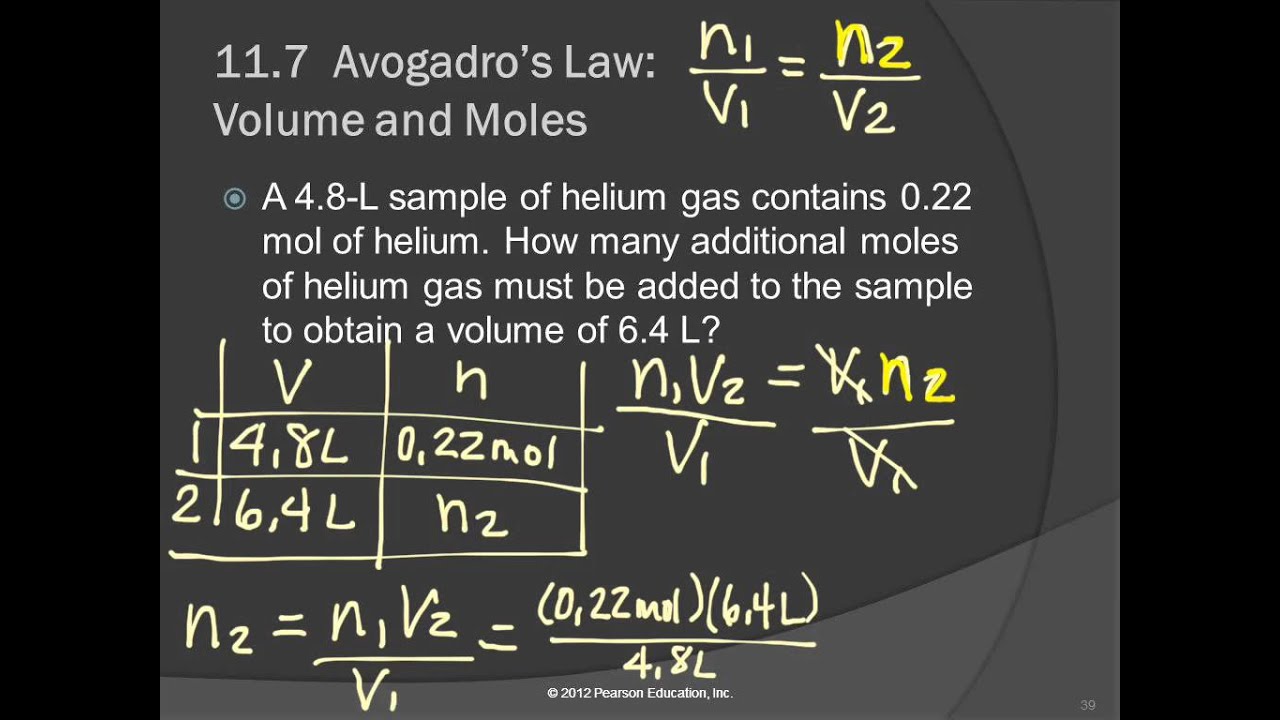
For a given mass of an ideal gas, the volume and amount (moles) of the gas are directly proportional if the temperature and pressure are constant.

The law is named after Amedeo Avogadro who, in 1812,^[2][3] hypothesized that two given samples of an ideal gas, of the same volume and at the same temperature and pressure, contain the same number of molecules. As an example, equal volumes of gaseous hydrogen and nitrogen contain the same number of atoms when they are at the same temperature and pressure, and observe ideal gas behavior. In practice, real gases show small deviations from the ideal behavior and the law holds only approximately, but is still a useful approximation for scientists.

Mathematical definition[edit]

The law can be written as:

${\displaystyle V\propto n}$

Illustrator cc for mac torrent. or

${\displaystyle \frac{V}{n}=k}$

where

V is the volume of the gas;

n is the amount of substance of the gas (measured in moles);

k is a constant for a given temperature and pressure.

This law describes how, under the same condition of temperature and pressure, equal volumes of all gases contain the same number of molecules. For comparing the same substance under two different sets of conditions, the law can be usefully expressed as follows:

${\displaystyle \frac{V_1}{n_1}=\frac{V_2}{n_2}}$

The equation shows that, as the number of moles of gas increases, the volume of the gas also increases in proportion. Similarly, if the number of moles of gas is decreased, then the volume also decreases. Thus, the number of molecules or atoms in a specific volume of ideal gas is independent of their size or the molar mass of the gas.

Derivation from the ideal gas law[edit]

The derivation of Avogadro's law follows directly from the ideal gas law, i.e.

${\displaystyle PV=nRT}$,

where R is the gas constant, T is the Kelvin temperature, and P is the pressure (in pascals).

Solving for V/n, we thus obtain

${\displaystyle \frac{V}{n}=\frac{RT}{P}}$.

Compare that to

${\displaystyle k=\frac{RT}{P}}$

which is a constant for a fixed pressure and a fixed temperature.

An equivalent formulation of the ideal gas law can be written using Boltzmann constantk_B, as

${\displaystyle PV=N{k}_{\mathrm{B}}T}$,

where N is the number of particles in the gas, and the ratio of R over k_B is equal to the Avogadro constant.

In this form, for V/N is a constant, we have

.

If T and P are taken at standard conditions for temperature and pressure (STP), then k′ = 1/n₀, where n₀ is the Loschmidt constant.

Historical account and influence[edit]

Avogadro's hypothesis (as it was known originally) was formulated in the same spirit of earlier empirical gas laws like Boyle's law (1662), Charles's law (1787) and Gay-Lussac's law (1808). The hypothesis was first published by Amadeo Avogadro in 1811,^[4] and it reconciled Dalton atomic theory with the 'incompatible' idea of Joseph Louis Gay-Lussac that some gases were composite of different fundamental substances (molecules) in integer proportions.^[5] In 1814, independently from Avogadro, André-Marie Ampère published the same law with similar conclusions.^[6] As Ampère was more well known in France, the hypothesis was usually referred there as Ampère's hypothesis,^{[note 1]} and later also as Avogadro–Ampère hypothesis^{[note 2]} or even Ampère–Avogadro hypothesis.^[7]

Experimental studies carried out by Charles Frédéric Gerhardt and Auguste Laurent on organic chemistry demonstrated that Avogadro's law explained why the same quantities of molecules in a gas have the same volume. Nevertheless, related experiments with some inorganic substances showed seeming exceptions to the law. This apparent contradiction was finally resolved by Stanislao Cannizzaro, as announced at Karlsruhe Congress in 1860, four years after Avogadro's death. He explained that these exceptions were due to molecular dissociations at certain temperatures, and that Avogadro's law determined not only molecular masses, but atomic masses as well.

Ideal gas law[edit]

Boyle, Charles and Gay-Lussac laws, together with Avogadro's law, were combined by Émile Clapeyron in 1834,^[8] giving rise to the ideal gas law. At the end of the 19th century, later developments from scientists like August Krönig, Rudolf Clausius, James Clerk Maxwell and Ludwig Boltzmann, gave rise to the kinetic theory of gases, a microscopic theory from which the ideal gas law can be derived as an statistical result from the movement of atoms/molecules in a gas.

Avogadro constant[edit]

Avogadro's law provides a way to calculate the quantity of gas in a receptacle. Thanks to this discovery, Johann Josef Loschmidt, in 1865, was able for the first time to estimate the size of a molecule.^[9] His calculation gave rise to the concept of the Loschmidt constant, a ratio between macroscopic and atomic quantities. In 1910, Millikan'soil drop experiment determined the charge of the electron; using it with the Faraday constant (derived by Michael Faraday in 1834), one is able to determine the number of particles in a mole of substance. At the same time, precision experiments by Jean Baptiste Perrin led to the definition of Avogadro's number as the number of molecules in one gram-molecule of oxygen. Perrin named the number to honor Avogadro for his discovery of the namesake law. Later standardization of the International System of Units led to the modern definition of the Avogadro constant.

Molar volume[edit]

Taking STP to be 101.325 kPa and 273.15 K, we can find the volume of one mole of gas:

${\displaystyle V_{\mathrm{m}}=\frac{V}{n}=\frac{RT}{P}=\frac{(8.314\text{J}\cdot {\text{mol}}^{-1}{\mathrm{K}}^{-1})(273.15\text{K})}{101.325\text{kPa}}=22.41{\text{dm}}^3{\text{mol}}^{-1}=22.41\text{liters}/\text{mol}}$

For 101.325 kPa and 273.15 K, the molar volume of an ideal gas is 22.4127 dm³⋅mol⁻¹.

See also[edit]

• Boyle's law – Relationship between pressure and volume in a gas at constant temperature
• Charles's law – Relationship between volume and temperature of a gas at constant pressure
• Gay-Lussac's law – Relationship between pressure and temperature of a gas at constant volume.
• Ideal gas – Mathematical model which approximates the behavior of real gases

Notes[edit]

1. ^First used by Jean-Baptiste Dumas in 1826.
2. ^First used by Stanislao Cannizzaro in 1858.

References[edit]

1. ^ ^abEditors of the Encyclopædia Britannica. 'Avogadro's law'. Encyclopædia Britannica. Retrieved 3 February 2016.CS1 maint: extra text: authors list (link)
2. ^Avogadro, Amedeo (1810). 'Essai d'une manière de déterminer les masses relatives des molécules élémentaires des corps, et les proportions selon lesquelles elles entrent dans ces combinaisons'. Journal de Physique. 73: 58–76.English translation
3. ^'Avogadro's law'. Merriam-Webster Medical Dictionary. Retrieved 3 February 2016.
4. ^Avogadro, Amadeo (July 1811). 'Essai d'une maniere de determiner les masses relatives des molecules elementaires des corps, et les proportions selon lesquelles elles entrent dans ces combinaisons'. Journal de Physique, de Chimie, et d'Histoire Naturelle (in French). 73: 58–76.
5. ^Rovnyak, David. 'Avogadro's Hypothesis'. Science World Wolfram. Retrieved 3 February 2016.
6. ^Ampère, André-Marie (1814). 'Lettre de M. Ampère à M. le comte Berthollet sur la détermination des proportions dans lesquelles les corps se combinent d'après le nombre et la disposition respective des molécules dont les parties intégrantes sont composées'. Annales de Chimie (in French). 90 (1): 43–86.
7. ^Scheidecker-Chevallier, Myriam (1997). 'L'hypothèse d'Avogadro (1811) et d'Ampère (1814): la distinction atome/molécule et la théorie de la combinaison chimique'. Revue d'Histoire des Sciences (in French). 50 (1/2): 159–194. doi:10.3406/rhs.1997.1277. JSTOR23633274.
8. ^Clapeyron, Émile (1834). 'Mémoire sur la puissance motrice de la chaleur'. Journal de l'École Polytechnique (in French). XIV: 153–190.
9. ^Loschmidt, J. (1865). 'Zur Grösse der Luftmoleküle'. Sitzungsberichte der Kaiserlichen Akademie der Wissenschaften Wien. 52 (2): 395–413.English translation.

Avogadro’s law tells about the relationship between the volume of a gas and the number of molecules possessed by it. It was formulated by an Italian scientist Amedeo Avogadro in the year 1811. During a series of experiments conducted by him, he observed that an equal volume of gases contains an equal number of particles. In other words, Avogadro’s law states that for an ideal gas, the volume of the gas is directly proportional to the number of moles possessed by it provided a constant temperature and pressure is maintained. This means that with an increase in volume, the number of moles will increase. Similarly, with a decrease in volume, the number of moles will also decrease. It is also known as Avogadro’s principle or Avogadro’s hypothesis. It is only applicable to ideal gases and gives an approximate result for the real gases. The gases with light molecules such as helium, hydrogen, etc. obey Avogadro’s law in a better manner as compared to the gases with heavy molecules.

Examples

1. Breathing

Avogadro's Law Is Expressed As

Human lungs demonstrate Avogadro’s law in the best possible way. When we inhale, the lungs expand because they get filled with air. Similarly while exhaling, the lungs let the air out and shrink in size. The change in volume can be clearly observed, which is proportional to the amount or the number of molecules of air contained by the lungs.

2. Inflating Balloon

To inflate a balloon it is required to be stuffed up with air molecules that are blown inside it either through the mouth or with the help of a pump. If you decrease the amount of air contained by the balloon, a significant decrease in the volume or the size of the balloon can be observed. Hence, Avogadro’s law can be observed in action.

3. Filling Tyres with Air

Flat tyres do not contain a sufficient amount of air molecules in them, which is why they lack proper shape. When the flat tyre of a vehicle is attached to an air pump, the air molecules get pressed into it. The number of particles of air present in the flat tyre increase; therefore, the volume increases accordingly. This helps the tyre to regain its original shape. Hence, the inflation of flat tyres is a clear demonstration of Avogadro’s law in everyday life.

4. Pumping Air in a Ball

A sports ball contains a bladder and a rigid outer covering. When the ball gets deflated the bladder gets deprived of air and loses its shape. Thereby, causing the ball to lose the ability to bounce. The volume of the air present in the bladder can be increased by forcefully pressing air into it through an air pump. The change in volume of air is proportionate to the change in the number of air molecules possessed by it. Hence, pumping air in a sports ball is an explicit illustration of Avogadro’s law in real life.

5. Bicycle Pump Action

Avogadro's Law Chemistry

The action of a bicycle pump makes use of Avogadro’s law. The pump extracts the air from the environment and pushes it inside the structure of the deflated object. The increase in the number of gas molecules in the object correspondingly changes its shape and helps it to expand. This proportionality between the number of molecules of the air and the volume is nothing but Avogadro’s law.

6. Pool Tube

How To Use Avogadro's Number

The pool tube, in deflated form, is easily portable in nature. The absence or scarcity of the number of molecules in the tube reduces the volume and makes it compact. During inflation, when the tube is filled with air, the number of air molecules inside it increases. Thereby, increasing the volume and size of the pool tube. Hence, Avogadro’s law can be implemented to inflate or deflate the pool tube as per the requirement.